最適化手法で生成された構造物の模型Structures Designed by Optimization Methods
計数工学科Department of Mathematical Engineering and Information Physics
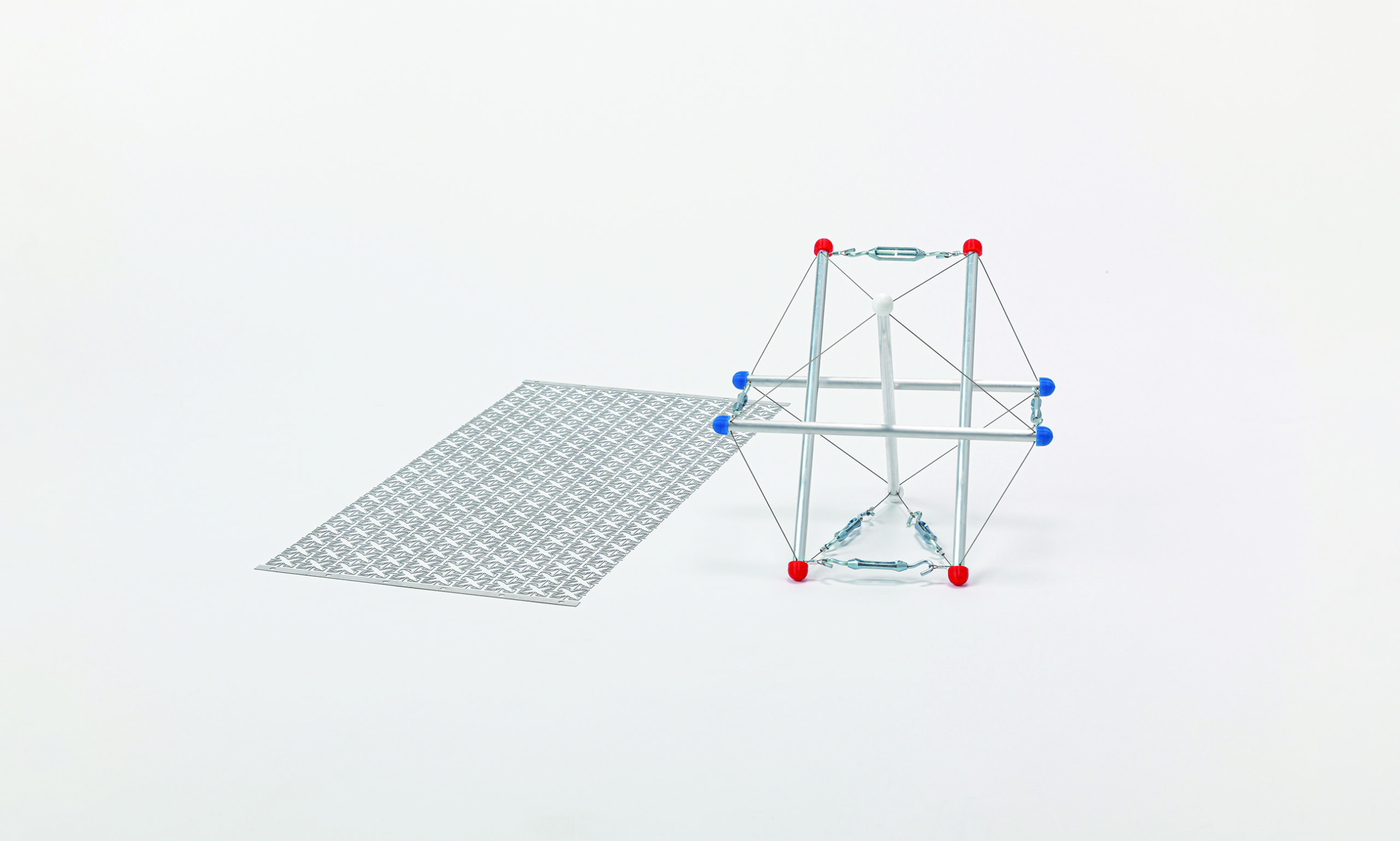
これらの模型は、最適化手法によって生成された構造物の例です。立体構造の方はテンセグリティとよばれるもので、平面構造の方は負のポアソン比をもつ周期構造です。テンセグリティは、張力と圧縮力が組み合わさった超安定な構造物であり、アメリカの建築家リチャード・バックミンスター・フラーによって「張力の海に浮かぶ圧縮力の島」と定義されました。これを満たすテンセグリティを新たに作り出すことは、容易ではありません。
一方、ポアソン比とは,物体をある方向に引っ張った伸ばした際に、その直交方向に縮む割合のことです。ほとんどの材料は正のポアソン比をもっていますが、形状を工夫することで負のポアソン比(ある方向に引っ張ると,その直交方向にも伸びる)を実現することが可能です。
このような特殊な物理的性質を有する構造物も、最適化(特に混合整数計画)を活用することで容易に設計することが可能となりました。
超安定なテンセグリティ
提供:東京大学 大学院工学系研究科 計数工学科 数理第5研究室
所蔵:東京大学大学院情報理工学系研究科 数理情報学専攻
負のポアソン比を有する周期構造
提供:東京大学 大学院工学系研究科 計数工学科 数理第5研究室
所蔵:東京大学大学院情報理工学系研究科 数理情報学専攻 寒野善博 教授
最適化手法によって生成された構造物の例: 超安定なテンセグリティ(立体模型)と負のポアソン比をもつ周期構造(平面模型)。
These are examples of structures designed by optimization methods. The three-dimensional model is a super-stable tensegrity composed of tension and compression components.
American architect Richard Buckminster Fuller defined it as an “island of compression in a sea of tension”. The two-dimensional model is a periodic structure with a negative Poisson’s ratio. Unlike conventional materials, when the structure is stretched in one direction, it expands in the perpendicular direction.
Large discrete structures with such special physical properties can now be designed by optimization methods (especially based on mixed integer programming).
A super-stable tensegrity
Courtesy: Mathematical Informatics 5th Lab, Department of Mathematical Engineering and Information Physics, Faculty of Engineering, The University of Tokyo
Collection: Department of Mathematical Informatics, Graduate School of Information Science and Technology, The University of Tokyo
A periodic structure with negative Poisson's ratio
Courtesy: Mathematical Informatics 5th Lab, Department of Mathematical Engineering and Information Physics, Faculty of Engineering, The University of Tokyo
Collection: Prof. Yoshihiro Kanno, Department of Mathematical Informatics, Graduate School of Information Science and Technology, The University of Tokyo
Structures designed by optimization methods: a tensegrity and a periodic structure with negative Poisson's ratio

